Golden Ratio and Vortices
Golden Ratio appears in vortices?
---
layout: post
read_time: true
show_date: true
title: "Golden Ratio in Vortices"
date: 2022-10-21
img: posts/20221021/point_vortex.jpg
tags: [Hydrodynamics, Dynamical System, Scientific Computation]
author: Hanchun Wang
description: "point vortex on the half plane"
---
Summary
Preview Version Published Version
In this two point vortices system on the half plane, we show that \(W\) is a non-dimensional parameter, and \(W=\phi, 1, \frac{1}{\phi}\) are three bifurcation values in this system. Where \(\phi\) is the Golden Ratio.
There are two mechanisms applied on a point vortex.
- Move along the boundary of the half plane
- rotate on the other vortex
These two mechanisms compete and lead to different types of the trajectories.
Vortex Pair


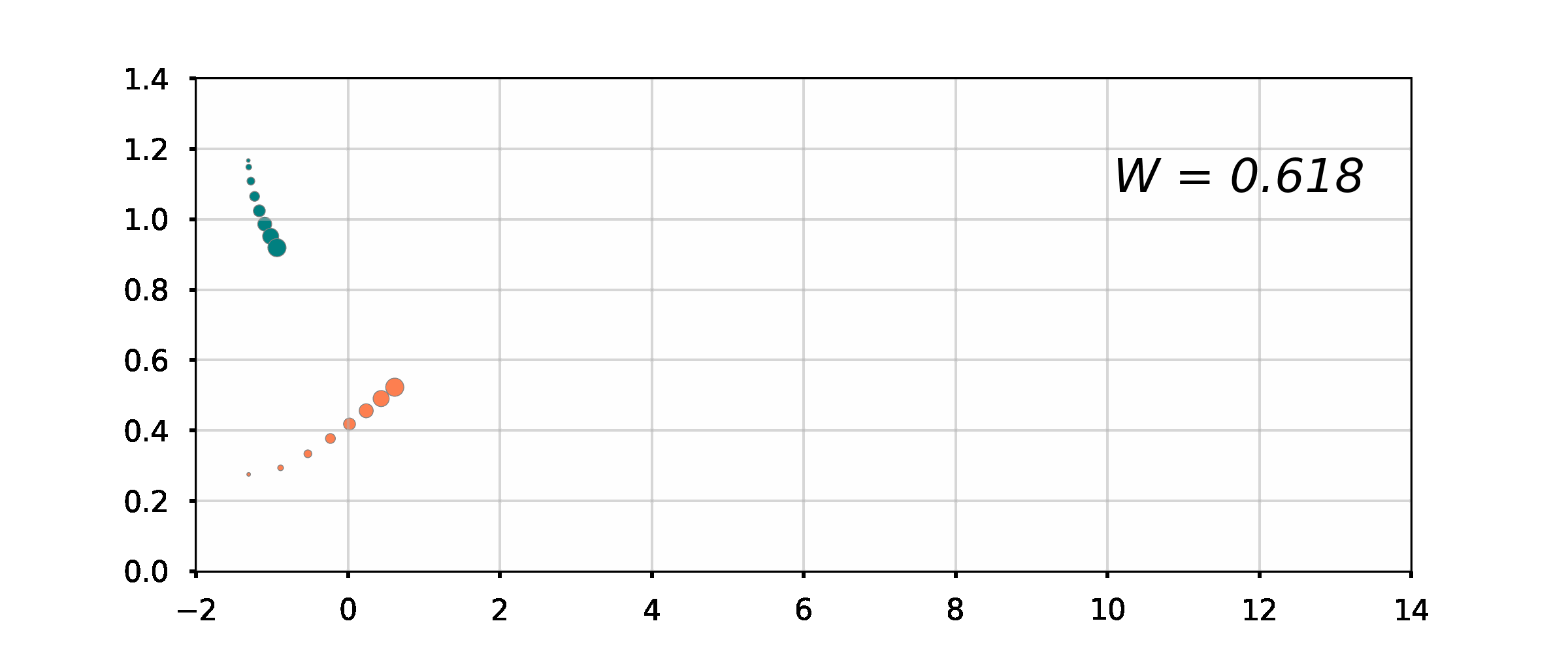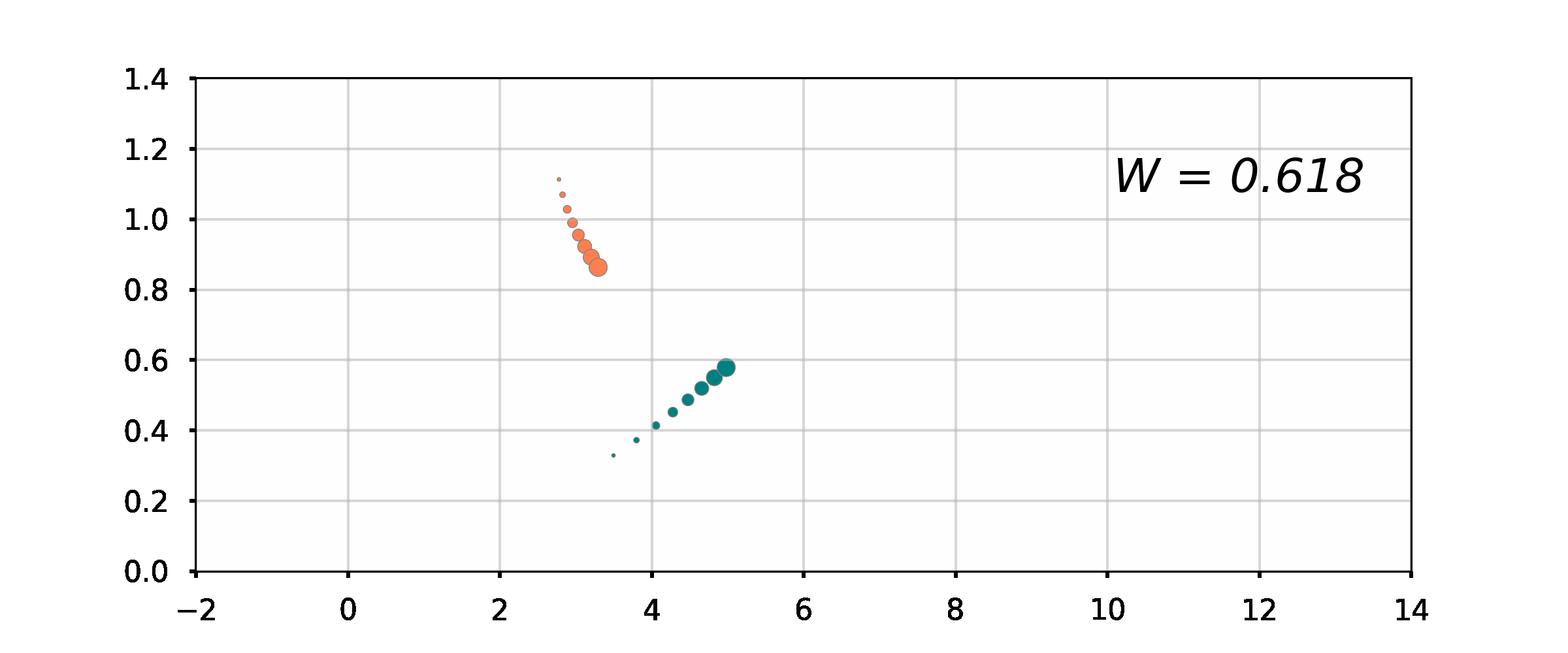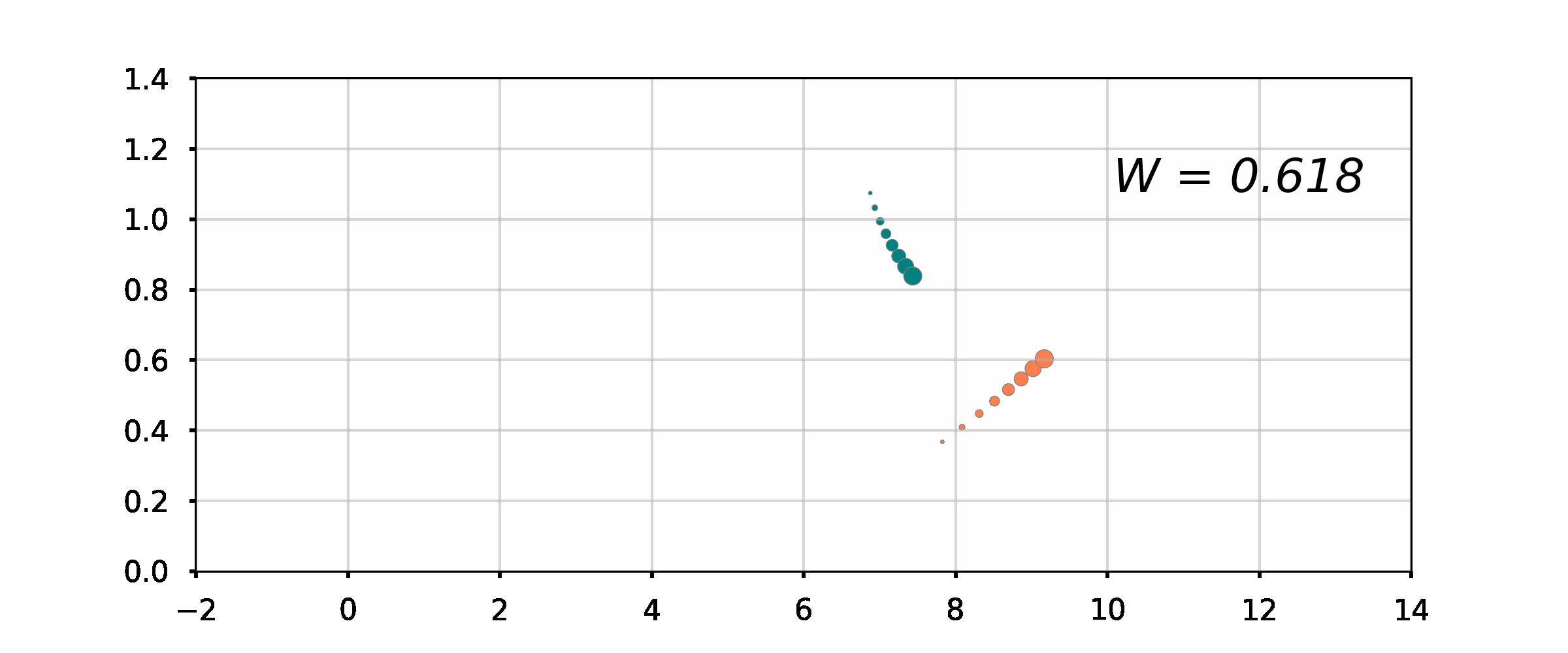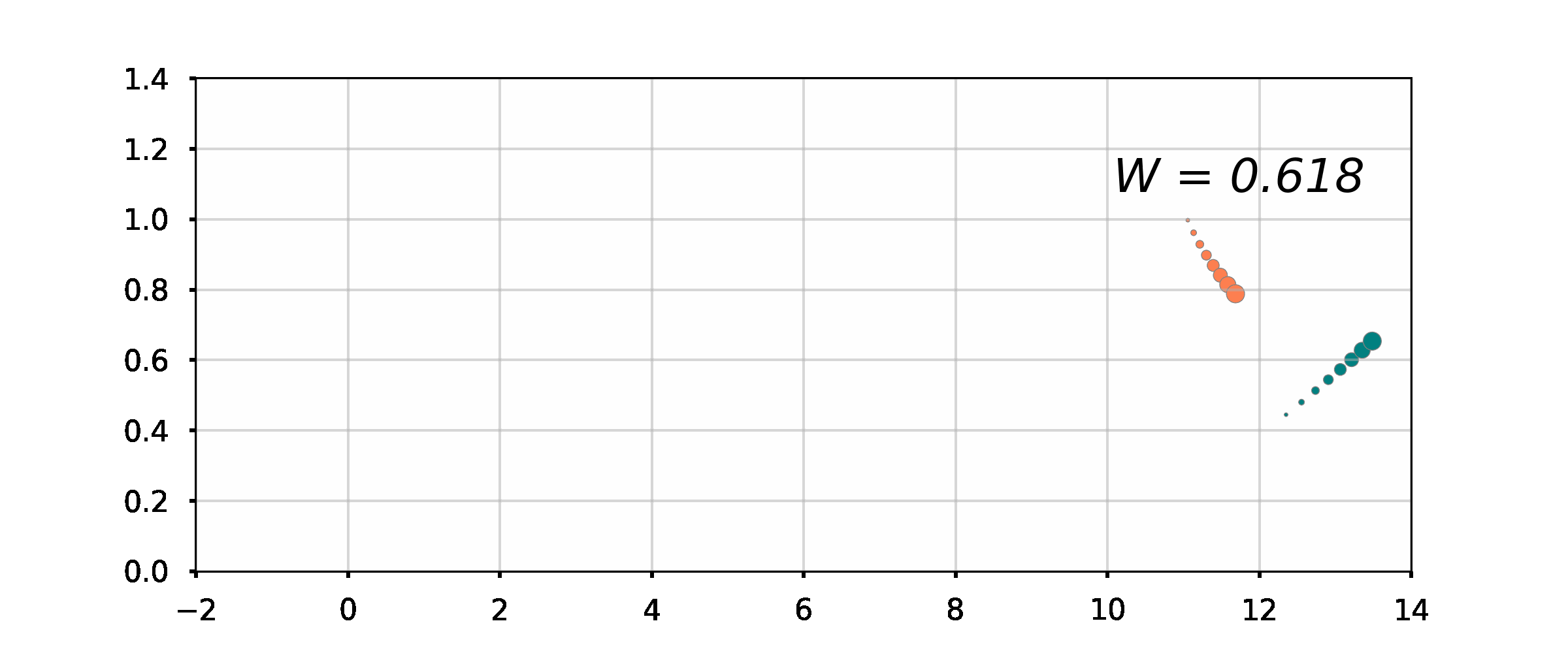
Vortex Dipole


Introduction
A single point vortex on the halfplane can be regarded as a vortex dipole that symmetric on the boundary \((y=0)\) in the full plane. Thus an \(N\) point vortex system in the halfplane is equivalent to a \(2N\) point vortex system in the full plane which are \(N\) point vortices and their \(N\) images.
The Green’s function on the half-plane \(\mathbb{R}_+^2\) is
\[G_{\mathbb{R}^2_+}\left( {z,z'} \right) = - \frac{1}{2\pi}\log ||z - z'||+\frac{1}{2\pi}\log ||z - z'^*||\]and the Hamiltonian is
\[H=\frac{1}{4 \pi} \log \left(\left(2 y_1\right)^{\Gamma_1^2}\left(2 y_2\right)^{\Gamma_2^2}\left(\frac{\left(x_1-x_2\right)^2+\left(y_1+y_2\right)^2}{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2}\right)^{\Gamma_1 \Gamma_2}\right)\]The first term represents the interaction between different vortices with their images; and the second term represents the interaction between a vortex and its image.
For the two point vortices system on the half plane, the Hamiltonian is The motion of equations are
\[\dot{x}_1=\frac{\Gamma_1}{4 \pi} \frac{1}{y_1}+\frac{\Gamma_2}{4 \pi}\left(\frac{2\left(y_1+y_2\right)}{\left(x_1-x_2\right)^2+\left(y_1+y_2\right)^2}-\frac{2\left(y_1-y_2\right)}{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2}\right)\]and
\[\dot{y}_1=\frac{-\Gamma_2}{4 \pi}\left(\frac{2\left(x_1-x_2\right)}{\left(x_1-x_2\right)^2+\left(y_1+y_2\right)^2}-\frac{2\left(x_1-x_2\right)}{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2}\right)\]To study vortex bifurcations we normalize their strengths by setting \(\Gamma_1=\Gamma_2=1\) for a vortex pair and \(\Gamma_1=-\Gamma_2=1\) for a dipole. Furthermore, introduce the following dimensionless parameter
\[W:=(P / \Gamma)^2 \exp \left(-4 \pi \mathcal{H} / \Gamma^2\right)\]measuring the vortex interaction where \(\Gamma:=\Gamma_1=\pm \Gamma_2\). As we will see below, the increase of \(W\) corresponds to the weakening of the interaction between the point vortices.
More GIF
Vortex Pair
When two point vortices have same strength, we have the following four scenarios.




Vortex Dipole
When two point vortices have opposite strength \(\Gamma_1=-\Gamma_2\), we have the following four scenarios.




